Skip to main content

SIMPLE HARMONIC MOTION: Definition, Equations, Particle Executing Simple Harmonic Motion

SIMPLE HARMONIC MOTION: DEFINITION, EQUATIONS, RELATIONS EXECUTING SIMPLE HARMONIC MOTION

What-is-Simple-harmonic-motion

What is Simple Harmonic Motion?

Simple Harmonic Motion (SHM) is defined as a to and fro of motion of an object about a mean position. The motion of a body is said to be simple harmonic if its acceleration is always,

a) directed towards a fixed point (mean position)

b) directly proportional to the distance from a fixed point.  

When a body is displaced in simple harmonic motion, a restoring force is set up. Due to this restoring force, the body comes back to its equilibrium, it gets kinetic energy and the body moves away from the equilibrium position. In this way, the body continuously oscillates back and forth about the mean position. Therefore, the motion is said to be a Simple Harmonic Motion (SHM).

Relation between acceleration and displacement of a particle executing simple harmonic motion (SHM):

Consider a particle having mass 'm' moving with a uniform circular motion with an angular velocity 'ω' in a circle of radius 'r' and center at 'O' as shown in the figure. If 'P' be the position of the particle at a time 't'. Draw 'P' perpendicular to OY then 'Q' is a foot of perpendicular drawn from point 'P' on the y-axis.

As the particle moves from point 'P' to 'R', 'Q' moves from point 'O' to point 'R' along the vertical diameter. When the particle moves from 'R' to 'S', 'Q' comes back from point 'R' to point 'O'. As the particle moves from point 'S' to point 'T', 'Q' moves from point 'O' to 'T' and when the particle moves from point 'T' to point 'A', 'Q' comes back from point 'T' to point '0' If the particle continuously goes around the circle, the projection 'Q' goes on vibrating to and fro about the center 'O' of the circle. 

Thus, the foot of perpendicular has to and fro motion along the diameter is simple harmonic motion (SHM).

Now from the shown figure,

Simple-Harmonic-Motion
sinθ = OQ / OP
OQ = OP sinθ 
i.e.  y = r sinθ ⇢ (i)
                where y is the displacement of point Q from the mean position.
y = r sin Ï‰t  [∵ Î¸ = Ï‰t ]
v = rω cos Ï‰t ⇢ (ii)

Further,
Simple-Harmonic-Motion-Equation
Simple-Harmonic-Motion-Equation
Simple-Harmonic-Motion-Equation
Simple-Harmonic-Motion-Equation⇢ (iii)   [∵ using equation (i)]

Now, acceleration is the rate of change in velocity
Simple-Harmonic-Motion-Equation

Equation (iv) is the equation relating the acceleration and displacement of a particle executing simple harmonic motion.
Thank You! for reading this Simple Harmonic Motion: Definition, Equations, Relations Executing Simple Harmonic Motion
If you really enjoyed reading then feel free to check our other blog:

If there are any other topics that you're confused about then you can suggest to us about it in the comment section so that we can clear your doubts easily and our next post might on that topic also.
Stay Safe, Stay Learning

Tags: simple harmonic motion, simple harmonic motion equation, simple harmonic motion definition, simple harmonic motion formula, a particle executes simple harmonic motion
Labels:

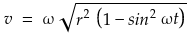
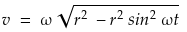
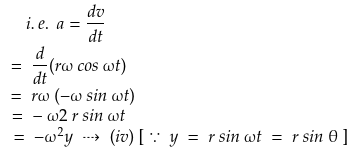
Comments

Post a Comment

Please, do not enter any spam links in the comment box.

Popular posts from this blog

SEE 2076 Routine- Secondary Education Examination 2076

SEE Examination Routine 2076 Finally, the Government of Nepal has published the routine of SEE Examination 2076 passed by the Ministry of Education. A few days ago, SEE Examination 2076 was said to be held in 10 th of Chaitra, 2076 but due to few circumstances, it was finalized for 6 th of Chaitra, 2076. Here down below is the routine of SEE Examination 2076 :  The Ministry of Education is expecting a lot better for this time as like previous year. All the best for your upcoming SEE Examination 2076 . If you're confused in anything than you can find it over this website or You may comment down below for whatever topic you need help for. Happy Study! Best Of Luck.
Post a Comment
Read more

Our Country Short Speech in English - Short Speech About Nepal

Our Country Short Speech in English Honorable Principle, Respected teachers and I wish my very good morning to all of my dear friends… Today, I am here in front of you all to deliver a very short and sweet speech about our country, Nepal. Our country Nepal is very well known among the globe. Most of the people from every nooks and corner of the world are known about our country. It is only possible because of the culture of our country, tradition, various religious and historical sites and the most important thing, the Mount Everest. We all know that Mount Everest which is the highest peak known in the world is located in Nepal and which makes Nepal to be known in the whole world. Another thing is that our country Nepal is very rich in culture and traditions. People living in Nepal might follow different cultures and traditions but there is not any activity of racism done in terms of culture and traditions. People living in Nepal are very kind, helpful and of go...
Post a Comment
Read more

Lewis Concept of Acid and Base: Limitations and Advantages

Lewis Concept of Acid and Base Lewis Acid : According to Lewis Concept, an acid is clearly defined as the substance which can accept a pair of non-bonding electrons. They are clearly known as an electrophile. They consist of vacant orbitals in their molecular structures. Ag + , Cu ++ and Fe +++ are the few examples of acid according to Lewis concept. There are various elements found which can act as Lewis acids. From the definition of Lewis acid, it is clearly known that all cations are Lewis acids since they are able to accept the pair of an electron (Cu 2+ , Fe 2+ , Fe 3+ ). Also, an ion, molecule, or an atom which is with an incomplete octet of electrons can act as a Lewis acid. Lewis Base : According to Lewis Concept, the base is defined as the substance which can donate the pair of non-bonding electrons. They are clearly known as a nucleophile. They consist of a lone-pair of electrons in their molecular structures. Cl - and OH - is an example of the base ...
3 comments
Read more